
where S n is the sum of the first n numbers, a 1 is the first number in the sequence, r is the common ratio of the sequence, and -1 < r < 1 for infinite series. There exists a formula that can add a finite list of numbers and a formula for an infinite list of numbers.
RECURSIVE FORMULA FOR GEOMETRIC SEQUENCE SERIES
Uizmaster: Finding Formula for General TermĪs we can see, the only difference between a sequence and a series is that a sequence is a list of numbers and a series is a sum of numbers. To find the 15th term, we will substitute 15 for the n-value, like this. We will now see how the explicit rule helps us to find the formula. The common ratio or r-value is 6 because we have to keep multiplying by 6 to go from one term to the next term. This means the formula is a n = 2 n-1 and the 9th term is 256.Įxample 2: Find the formula for sequence B, seen below, and use it to determine the 15th term in the sequence. To find the 9th term, we would simply plug in 9 for the n-value and get this. Now we use the explicit rule to gain a formula, like so. The fixed number, called the r-value or common ratio, is 2.

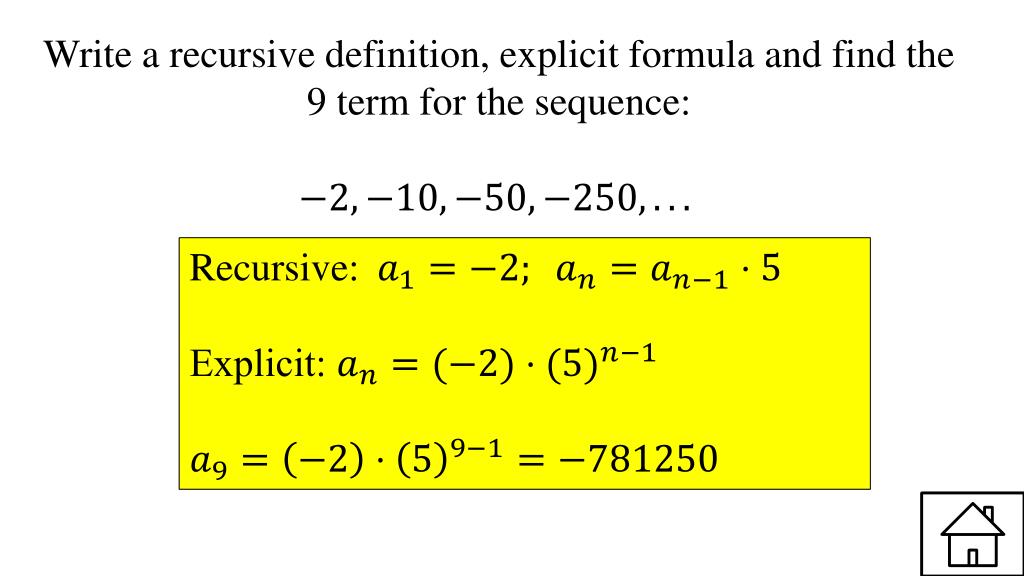
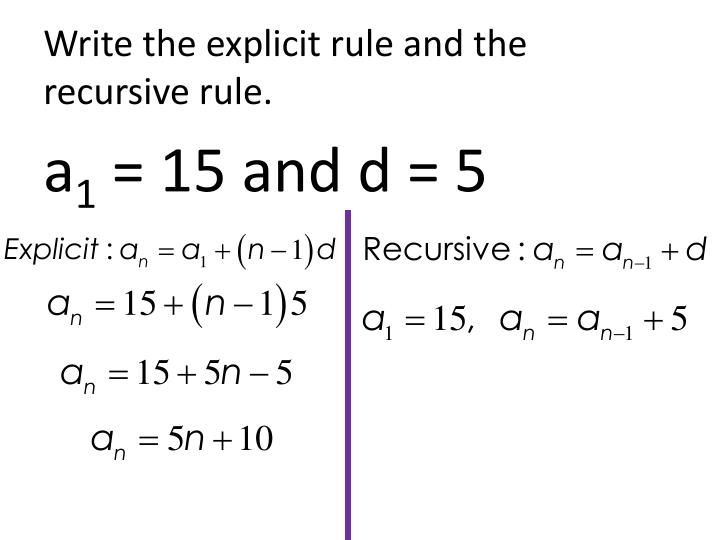
We will look at two examples to explain this skill.Įxample 1: Calculate the formula for sequence A, seen below, and use it to find the 9th term in the sequence. We will use the explicit rule to help us. In this section, we will look at coming up with a unique formula to define all the terms in a geometric sequence. As can be seen, the explicit rule is far superior to the recursive rule. This means the 20th term is equal to 11,622,615. To find the 20th number, all we have to do is multiply the first number in the sequence by the common ratio raised to the 19th power, like this. What if we wanted to find the 20th number? We would have to find all off the numbers before the 20th number, if we use the recursive rule. If we multiply the 5th term by 3 we get the 6th term, which is 243, and so on. If we wanted to find the 5th term, we would multiply the 4th term by 3 to get 81. We can see that the common ratio is 3 because we have to keep multiplying by 3 to go from one term to the next. Let us say we were given this geometric sequence. The recursive rule means to find any number in the sequence, we must multiply the common ratio to the previous number in this list of numbers. To determine any number within a geometric sequence, there are two formulas that can be utilized. where n is any positive integer greater than 1. The r-value, or common ratio, can be calculated by dividing any two consecutive terms in a geometric sequence. This notation is necessary for calculating nth terms, or a n, of sequences. This means that if we refer to the tenth term of a certain sequence, we will label it a 10. Mathematicians also refer to generic sequences using the letter a along with subscripts that correspond to the term numbers as follows: Mathematicians use the letter r when referring to these types of sequences. So that we can examine these sequences to greater depth, we must know that the fixed numbers that bind each sequence together are called common ratios.
The fourth number times -1/2 is the fifth number: -2 × -1/2 = 1.īecause these sequences behave according to this simple rule of multiplying a constant number to one term to get to another, they are called geometric sequences. This too works for any pair of consecutive numbers. We need to multiply by -1/2 to the first number to get the second number. Sequence C is a little different because it seems that we are dividing yet to stay consistent with the theme of geometric sequences, we must think in terms of multiplication.
The third number times 6 is the fourth number: 0.36 × 6 = 2.16, which will work throughout the entire sequence. This also works for any pair of consecutive numbers. The second number times 2 is the third number: 2 × 2 = 4, and so on.įor sequence B, if we multiply by 6 to the first number we will get the second number. This works for any pair of consecutive numbers. įor sequence A, if we multiply by 2 to the first number we will get the second number. The following sequences are geometric sequences:

Algebra 2 honors practice test.Sequences of numbers that follow a pattern of multiplying a fixed number from one term to the next are called geometric sequences.


 0 kommentar(er)
0 kommentar(er)
